2PPE Examples

Examples

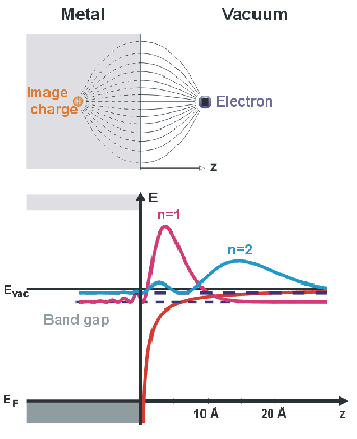
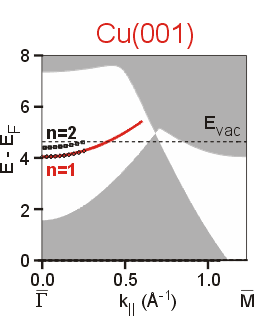
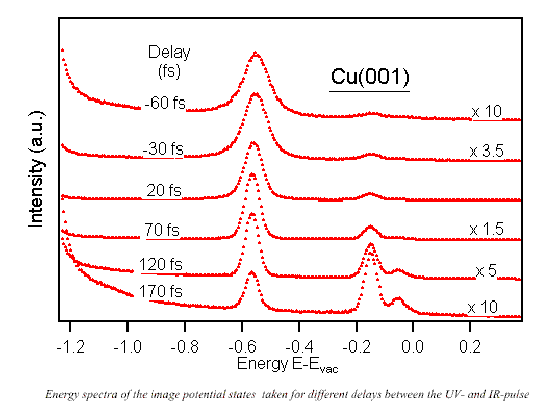
Image-Potential States

The force an electron feels is in front of a conductive surface at a distance z is equivalent to the force between two electrons with opposite charges separated by a distance 2z. The corresponding attractive image potential is therefore given by

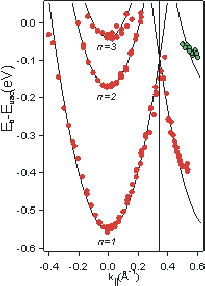
If it is unlikely that the electron penetrates into the bulk due to a band gap, bound states will be formed and the potential results in energy states similar to these of a hydrogen atom:

The quantum defect a arises because of the finite probability of the electron penetrating in the bulk.

In the figure on the left you can see the projected bulk band structure of Cu(001). The vacuum level is in the middle of the band gap. This leads to the image-potetnial states. In the figure below several energy spectra are plotted fot different delays. A positive delay in this context means that the probe pulse (IR pulse) follows the UV pulse. You can see that for positive delay the n=1 state (at about 0.54eV) has nearly vanished whereas the n=2 and n=3... states are still comparatively strong. This can be explained by the much longer lifetime of these states.

Dispersion

The wave vektor (k) of bulk and surface states can be obtained by angular-resolved measurements. While the k_|| component is always preserved in photoemission the change of the

vector of the photoelectrons has to be considered in the spectroscopy of bulk states.

This problem does not arise, if you look at surface states, since no

is defined for these 2D objects. The energy dispersion of the detected photoelectron equals the dispersion relation of a free electron

,
whereas the k-vector can be divided in its parallel and perpendicular components:

.
So, the relation between the detection angle q and the k_|| component in the corresponding direction is given by

A Cu(001) surface with a miscut of 11° is designated as Cu(117). The quite regular arrangement of the terraces (see figure) leads to a rectangular surface unitcell with a length of seven atomic distances including two steps. Thus the corresponding Brillouin zone is seven times smaller perpendicular to the steps, than the Brillouin zone of Cu(001). In the figure below you can see the dispersion spectrum of Cu(117). The umklapp of the image-potential states at the border of the Brillouin zone is clearly visible.

Lifetime

When the pulse width is small it is possible to see the exponential decaying rate

of an image-potential state. So time-resolved measurements can directly yield the lifetime.

Quantum-Beat Spectroscopy

Since we have ultra-short pulses the spectral width of the laser pulses is not always negligible. For example, a pulse width of t=95fs corresponds to an energy width of DE=14meV. This leads to an interesting phenomenon if two energy states n and n+1 are populated by a pulse which width is larger than the energy difference of these two states. In this case these two levels are populated coherently. The coherent excitation of each of these levels gives the following wavefunctions:

.
The 2PPE intensity is proportional to the square of the superposition of these two wavefunctions

.
The frequency of the beating term, which modulates the exponential decay is

These quantum beats can be seen in time-resolved 2PPE on image-potential states with n>2. It can be used to extract the energy of the states which can't be resolved in the energy spectrum anymore.
Quantumbeat Spectrum