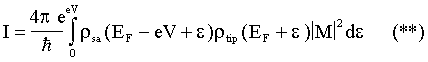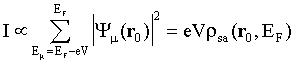2. Theory
2.1. Electron Tunneling
In classical physics an electron cannot penetrate into or across a potential
barrier if its energy E is smaller than the potential
 within the barrier.
A quantum mechanic treatment predicts an exponential decaying
solution for the electron wave function in the barrier. For a rectangular barrier we get
within the barrier.
A quantum mechanic treatment predicts an exponential decaying
solution for the electron wave function in the barrier. For a rectangular barrier we get
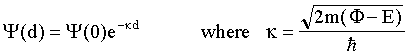 .
.
The probability of finding an electron behind the barrier of the width d is
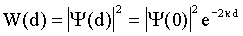 .
.
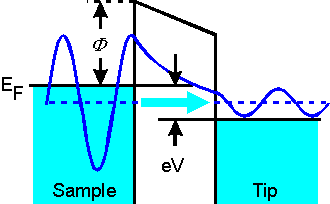
In scanning tunnneling microscopy a small bias voltage V is applied so that due
to the electric field the tunneling of electrons results in a tunneling current I.
The height of the barrier can roughly be approximated
by the average workfunction of sample and tip.
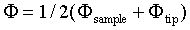
If the voltage is much smaller than the workfunction
eV <<  ,
the inverse decay length for all tunneling electrons can be
simplified to
,
the inverse decay length for all tunneling electrons can be
simplified to
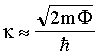 .
.
The current is proportional to the probability of electrons to tunnel through
the barrier:
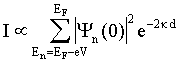 .
.
By using the definition of the local density of states for 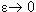
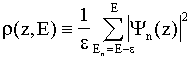
the current can be expressed by
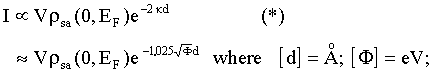 .
.
With 5eV as typical example for a workfunction value a change of 1Å
in distance causes a change of nearly one order of magnitude in current.
This facilitates the high vertical resolution.
Also
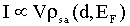 ,
,
which means that the current is proportional to the local density of states of
the sample at the Fermi energy at a distance d, i.e. the position of the tip.
A more exact calculation of the current density of the square barrier problem
requires the Schrödinger's equation to be solved in the three regions:
before, in and behind the barrier.
The coefficients have to be adapted so that the overall solution is continually
differentiable. Defining the transition probability as
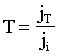
yields

with the approximation
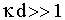 .
.
The current density itself is defined as
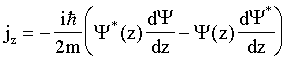 .
.
For a nonsquare potential the WKB method must be used. This is more adequate
as the potential is changed by the applied voltage and influenced by the
image force on the electron. The WKB method yields a transition probability of
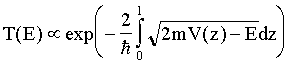 .
.
2.2. Bardeen Approach
Another way of describing electron tunneling comes from Bardeen's
approach which makes use of the time dependent perturbation theory.
The probability of an electron in the state
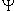 at
at 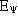 to tunnel into a state
to tunnel into a state
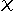 at
at
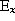 is given
by Fermi's Golden Rule
is given
by Fermi's Golden Rule
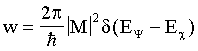
The tunneling matrix
element is given by an integral over a surface in the barrier region lying
between the tip and the sample:
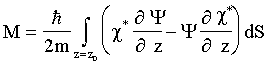 .
.
Applying a bias voltage V and approximating the Fermi distribution as a step function
(kT  Eresolution), the current is
Eresolution), the current is
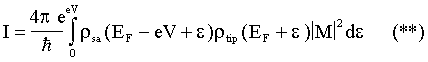
Hence the current is given by a combination of the local densities of states of
the sample and the tip, weighted by the tunneling matrix element M.
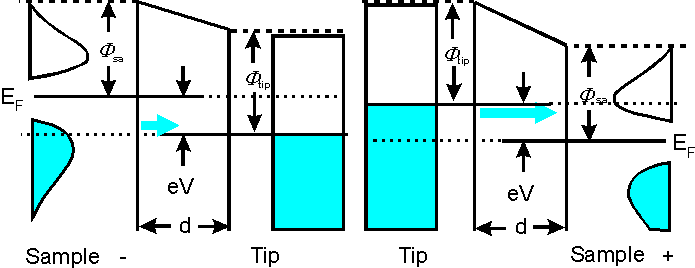
Schematic of electron tunneling with respect to the density of states of the sample.
|
 means that an electron can only tunnel if there is an unoccupied state
with the same energy in the other electrode (thus inelastic tunneling is not
treated). In case of a negative potential on the sample the occupied
states generate the current, whereas in case of a positive bias the unoccupied
states of the sample are of importance.
Therefore, as shown below, by altering the voltage, a complete
different image can be detected
as other states contribute to the tunneling current.
This is used in tunneling spectroscopy.
It should finally be mentioned that the probability of tunneling
(expressed by M2)
is larger for electrons which are close to the fermi edge due to the lower
barrier.
means that an electron can only tunnel if there is an unoccupied state
with the same energy in the other electrode (thus inelastic tunneling is not
treated). In case of a negative potential on the sample the occupied
states generate the current, whereas in case of a positive bias the unoccupied
states of the sample are of importance.
Therefore, as shown below, by altering the voltage, a complete
different image can be detected
as other states contribute to the tunneling current.
This is used in tunneling spectroscopy.
It should finally be mentioned that the probability of tunneling
(expressed by M2)
is larger for electrons which are close to the fermi edge due to the lower
barrier.

Imaging the occupied states of
SiC(000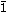 )3x3 )3x3 |

Imaging the unoccupied states of
SiC(000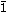 )3x3 )3x3 |
2.3. Lateral resolution
The lateral resolution of STM can not be understood in terms of a
Fraunhofer diffraction resolution.
The corresponding wave length of the tunneling electron would be
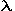 > 10 Å.
> 10 Å.
Therefore the STM works in the near-field regime.
The overall geometric curvature of the tip with a radius of curvature of
e.g. 1000 Å and
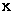 = 1 Å-1
would give rise to a resolution of about 50 Å.
= 1 Å-1
would give rise to a resolution of about 50 Å.
The actual atomic resolution can only be understood in a quantum mechanical view:
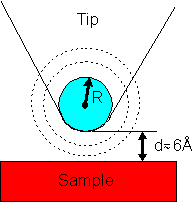
The most prominent model in this respect is the s-wave-tip model.
The tip is regarded as a protuding piece of Sommerfeld metal with a Radius of
curvature R (see Figure).
It is assumed that only the
s-wave solutions of this quantum mechanical problem (spherical potential
well) are important. Thus, at low bias the tunneling current is proportional to the local
density of states at the center of cuvature of the tip r0:
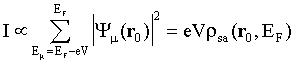
In this model only the properties of the sample contribute to the STM image
which is quite easy to handle. But it cannot explain the atomic resolution. |
 |
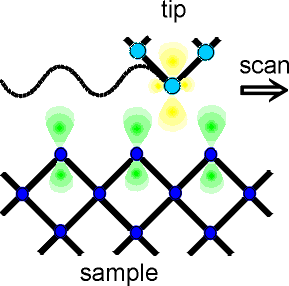
Interaction which causes a high corrugated tunneling distribution |
Calculations and experiments showed that there is often a dz2
like state near the fermi edge present at
the apex atom which also predominantely contributes to the tunneling current.
It is understood that this state (and also the pz like state)
is advantageous for a „sharp„ tip.
Since the tunneling current is a convolution of the tip state and the sample state,
there is a symmetry between both: By interchanging the electronic state of the tip and the sample
state, the image should be the same (reciprocity principle). This can also explain the fact
that the corrugation amplitude of an STM image is often larger than that of
the LDOS of the sample (measured by helium scattering). In this case the tip traces a fictitious
surface with a dz2 like state.
The state of
the tip atom is dependent on the material and the orientation. As the tip is quite difficult
to handle, it is one of the most difficult problems in a STM experiment. |

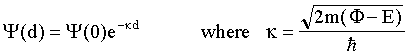 .
.
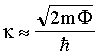 .
.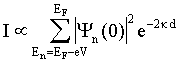 .
.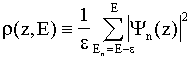
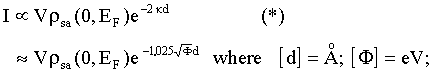 .
.
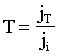

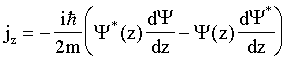 .
.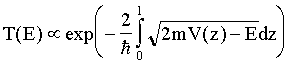 .
. 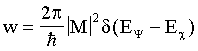
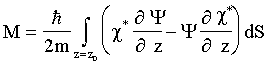 .
.